PreAmble: Newton (1643 - 1727) & Faraday (1791 - 1867)
- Electric field (Previous Lecture)
- Particle has (q, m) and a {observables}
- Newton: a = ΣF / m (F includes contact and non-contact forces)
- ΣF = Fcontact + Fg + Fe + ...
- Faraday: For long range forces, better to think of fields as intermediary (like talk-air-ear). On earth surface: ΣF = Fcontact + m g + q E + ...
Key Points: Electric (Gravitational) Fields
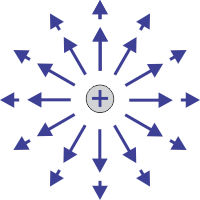
Point Charges & Symmetry
For Macroscopic objects we need to (Σ) or ∫ over all q (electric) or m (gravity). Generally this is done numerically. But there are a few simple cases...
| 4 Simple Cases | |||
|---|---|---|---|
| $$ \mathbf E_{point} $$ | $$ \mathbf E_{line,\infty} $$ | $$ \mathbf E_{plane,\infty} \, $$ | $$ \mathbf E_{sphere} $$ |
| 
| 
| |
| $$ q \, [C] $$ | $$ \lambda \, [C m^{-1}] $$ | $$ \eta \, [C m^{-2} ] $$ | $$ Q \, [C] \, $$ |
| $$ {1 \over {4 \pi \epsilon_o}} \, \color{fuchsia} {{1 \over r^2} \, q} \, { \mathbf {\hat r} } $$ | $$ {1 \over {4 \pi \epsilon_o}} \, \color{fuchsia}{{ 2 \over r} \, \lambda } { \mathbf {\hat r} } ={1 \over {2 \pi \epsilon_o}} \, \color{fuchsia}{{ 1 \over r} \, \lambda } \, { \mathbf {\hat r} } $$ | $$ {1 \over {4 \pi \epsilon_o}} \, \color{fuchsia}{2 \pi \, \eta } \, { \mathbf {\hat z} } = {1 \over {2 \epsilon_o}} \, \color{fuchsia}{\eta } \, { \mathbf {\hat z} }$$ | $$ = \mathbf E_{point} $$ |
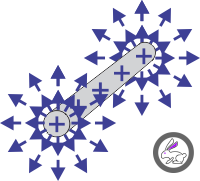
Dipoles
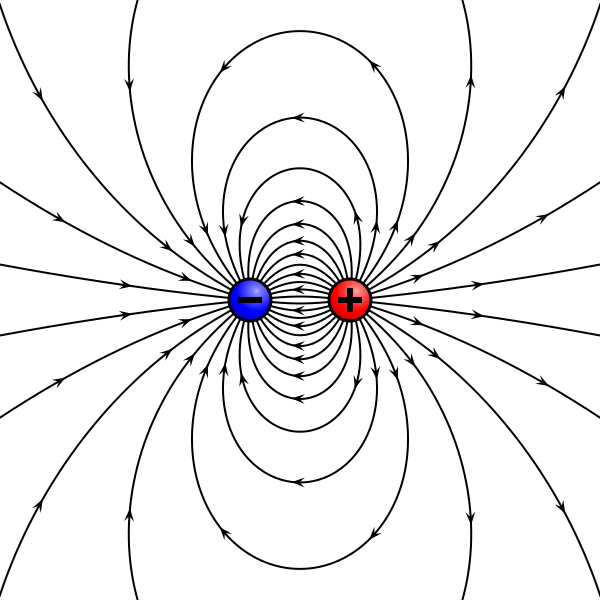
| $$ \mathbf p = |q| \, \mathbf {s} \, $$ | |
|---|---|---|
| $$ \mathbf E_{dipole,axis} $$ | $$ \mathbf E_{dipole,plane} $$ | |
| $$ = {1 \over {4 \pi \epsilon_o}} \, \color{fuchsia} {2 \mathbf p \over r^3} $$ | $$ = {1 \over {4 \pi \epsilon_o}} \, \color{fuchsia} { \mathbf p \over r^3} $$ | |
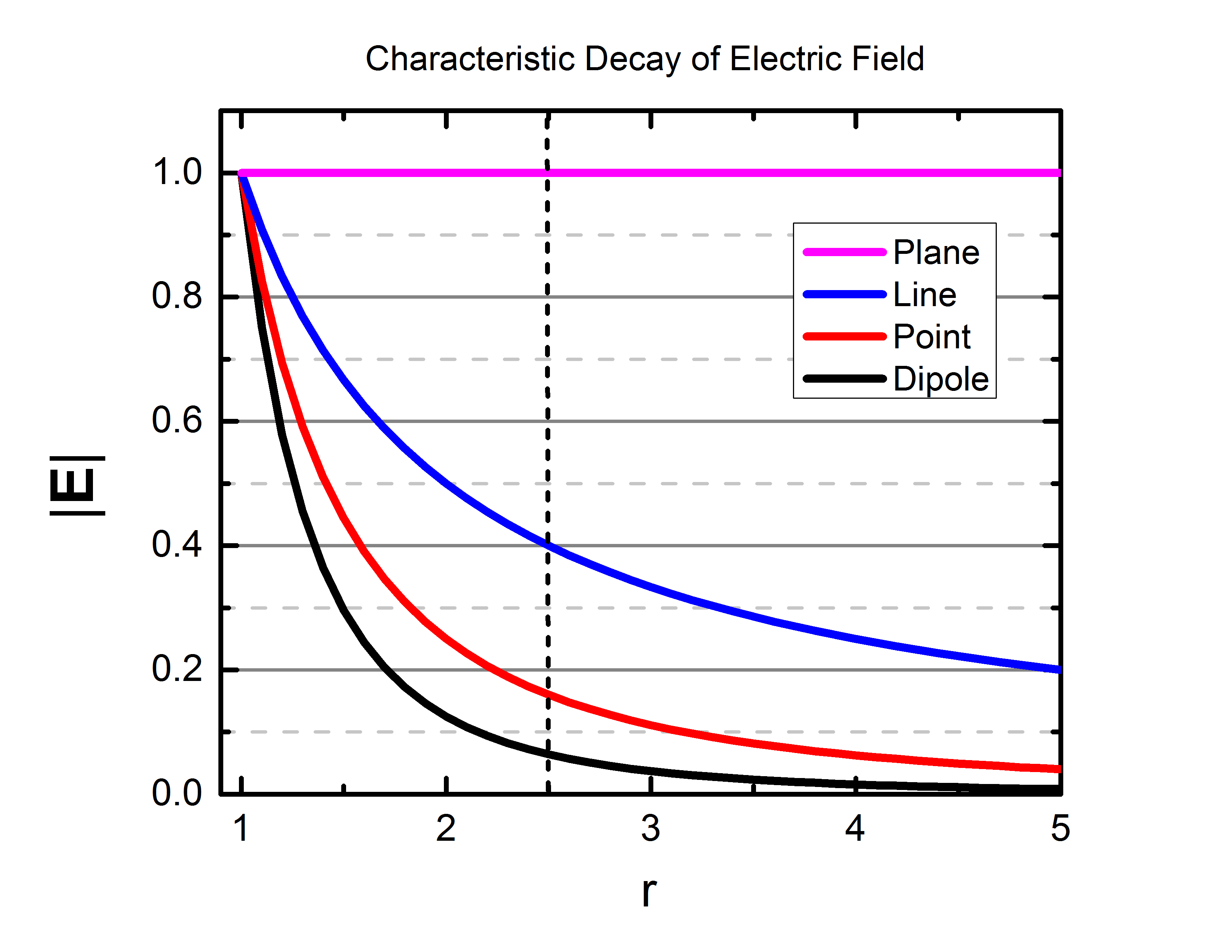
Decay of Electric Field by type
(Observable) Acceleration: Linear & Angular
| $$ \mathbf a = { q \over m_e} \mathbf E + { m_g \over m_e} \mathbf G $$ | $$ \mathbf \alpha = {{\mathbf \tau} \over {I}} = {{\mathbf p \times \mathbf E} \over I} $$ |
Powerpoint & Kahoot
1 Review by Kahoot
2 Electric Dipole: intro then ppt23-31 to 42
3 Continous Charge Distributions - Line of Charge 43-54
4 Continue Charge Distributions - Rings/Disks/Planes/Spheres 55-63
5 Parallel Plate Capacitor 64-73
6,7 Motion in Electric Fields 74-100
Charged particle
Dipole (no charge, ΣFe=0 but the field does something....)
