← (ne10) Current & Resistance 電流和電阻 →

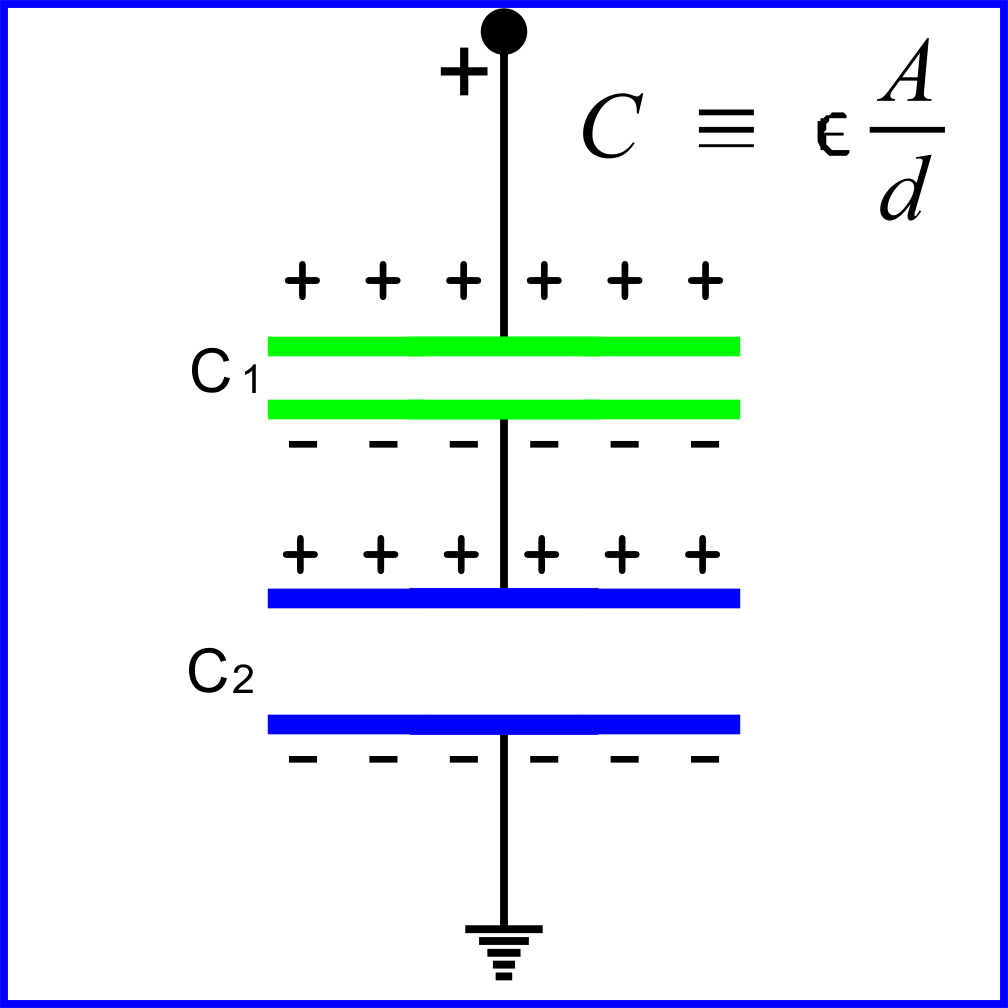
Review: Capacitors ΔV = Q/C (finding equivalent capacitor: Q, ΔV don't change)

Key Points

Current Density

$$ { \vec J = \, n \, e \, \vec v_d \, = \, n \, e \, \left( { {e \tau} \over m} \vec E \right) \equiv \sigma \vec E \equiv {1 \over \rho} \vec E \, \,\, [Am^{-2}] }\\ $$

Ohm's Law

From Basic Physics with	Assumptions
$$ I \equiv \iint \vec J \cdot d \vec A = {1 \over \rho} \iint \vec E \cdot d \vec A $$	ρ≠f(x,y,z) → drift velocity linearly (only) depends on E-field (τ = C)
$$ I = {1 \over \rho} \iint \vec E \cdot d \vec A = {1 \over \rho} \vec E \cdot \iint d \vec A $$	E is constant across the conductor cross section
$$ I = {1 \over \rho} \vec E \cdot \vec A = {1 \over \rho} {\Delta V \over L} A $$	E is ⊥ to conductor cross section. E is constant along wire.
$$ \rightarrow I = {A \over {\rho L}} \Delta V \equiv {{\Delta V} \over R}\\ $$

Kirchoff's Laws

1: Energy Conservation	$$ \Delta V_{closed \, loop} = \sum \Delta V_i = 0 \, \\$$
2: Charge Conservation	$$ \sum I_{in} - \sum I_{out} = 0 \\$$

Other

• Nonuniform Charge Distribution along surface of wire   →   longitudinal E-Field in wire   →   charge flow (current) in wire
• Battery is the source of everything: ℰ = W/q
  → ΔV_bat = (1 - δ) ℰ (internal resistance)
  → Δ V_wire
  → E_wire = Δ V_wire/L
  → I = σ A E_wire = A/ρ E_wire
  Magnitude of current is related to both battery's ℰ and wire's resistance: I = ΔV_bat/R_wire

PBL Comments

Lecture Power Points: Current (J & I) and Resistance (R)

Knight Chapter 27